Authors: Bart Muys, Jan den Ouden, Kris Verheyen, Frits Mohren
Level: basic knowledge / BSc
14.1 Introduction
Growth is the increase in biomass and in the dimensions of a tree or stand. Through cell division and cell stretching in meristem tissues, conversion of sugars produced during photosynthesis into structural biomass leads to an increase in size, and to growth of the tree. The biomass thus produced is allocated to leaves or needles, branches, stem and roots. In the top meristem, this results in height growth; in the cambium this leads to growth in thickness of the stem, mainly due to the formation of xylem tissue (see Chapter 12). The allocation of biomass according to a morphogenetic growth pattern (see 12.1) determines the habitus (shape) of the tree. Tree growth is the driving force behind forest development, and understanding of growth in all its aspects is crucial.
In the first growth phase a tree primarily invests in height growth (primary growth), and later on, thickness growth becomes dominant (secondary growth). From an evolutionary perspective, this can be explained by the fact that a tree has an advantage in first bringing its leaves into a favourable position to intercept sunlight, i.e. above the surrounding trees. Next, the tree needs to develop a solid stem to maintain the acquired position in the canopy and to carry the increasing weight of the developing crown.
The growth of a tree depends on its genetic predisposition (species or origin; see chapter 17), temperature and radiation, and the availability of water and nutrients (site quality; see chapter 18). Within a forest stand with homogeneous site conditions, where moisture and nutrients are in ample supply, individual tree growth will be primarily depending on available light, and will therefore be proportional to the tree’s share of light interception. The consequences of this for social interaction with neighbouring trees (e.g. competition) are further elaborated in Chapter 16. Here, only global patterns in height, diameter and volume growth are described, and root growth is briefly discussed, including variation in growth due to differences in site quality.
14.2 Height growth
Height growth results from cell division and cell stretching in the top meristem, located in the terminal bud of the main axis of the tree (see 12.7.1). Depending on the genetically determined branching pattern in interaction with environmental factors, tree shapes develop differently. Monopodial growth occurs in tree species where a single continuous growth axis extends its apex and produces successive lateral shoots. It will either result in a monocormic crown consisting of a central straight stem (monopodium) with branch whorls around, like in most conifers, or a polycormic crown where the central stem present at the base gets dissolved in a branched crown with irregular structure, like in monopodial broadleaved like maples or ashes (fig. 14-1). Sympodial growth occurs in tree species where the main axis is made up of successive secondary axes, like in oaks and beech trees. They always result in polycormic crowns (fig 14.1).Sympodial growth is advantageous from an evolutionary point of view, because it offers the flexibility to position leaf tissue at any point of the three-dimensional crown space and thus may maximise the use of available light. But when forest management focuses on timber production, the monopodial architecture of the conifers is often preferred, because long straight stems with whorls of side branches are easier to harvest, to delimb, and process mechanically.
When a tree grows within a forest, the upper crown parts shade the lower living branches, causing them to die due to lack of light. Due to the loss of dead branches (also called self-pruning) and continued diameter growth, a straight branch-free bole may eventually develop below an otherwise branched tree crown. In general self-pruning is more common in broadleaved than in conifers, which is why conifers more often receive stem-raising pruning interventions (see chapter 43). Higher up along the stem, green branches remain alive because they have their leaves in the light-exposed parts of the crown, so that they continue to be supplied with assimilates and do not die.

Figure 14-1: Different tree shapes resulting from different branching patterns. On the left a Scots pine, with a clear monopodial growth and strong apical control, creating a monocormic stem and crown. In the middle a common ordinary ash with monopodial growth but weaker apical control and regular mortality of the apex creating a polycormic crown. On the right a hornbeam with sympodial growth: mortality of branches as a result of shading (and pruning) has created a monocormic stem base, but higher along the stem a polycormic crown has formed without a clear central axis (see also 12.7). Photos Leo Goudzwaard.
Height growth exhibits a daily (diurnal) periodicity that differs between species. Some species grow mainly at night, while others show a period of rapid growth during the day and a period of slow growth or rest during the night (Mitscherlich 1978; fig. 14-2). Furthermore, daily height growth depends on temperature and moisture availability. Seasonality in height growth may also vary between tree species (see fig. 14-3). Species with an outspoken rhythmic height growth, such as oak, beech, pine, spruce and silver fir, have a short concentrated height growth period of only 4-6 weeks in the spring, while other species continue to grow in height for most of the growing season, such as hornbeam, poplar, willow, birch, black locust, douglas fir and larch. In years with favourable weather conditions, especially species of the first group may form a second or even a third growth shoot in the summer (see box 14-1: Lammas shoots).
The daily height growth of a tree is in the order of millimetres to centimetres; the annual increase in height in the order of decimetres to meters.
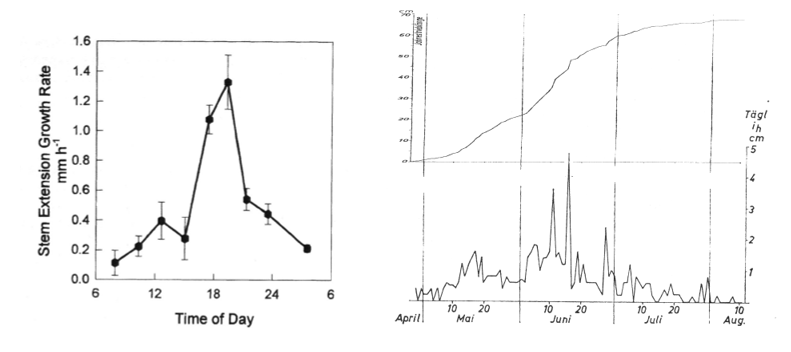
Figure 14-2: The course of height growth during the day and over the growing season. Left: Typical diurnal pattern of height growth in peach (Prunus persica) growing in optimal conditions. Each point is the average of 5 top shoots. After Berman & De Jong (1997).
Right: Height growth of a douglas fir during the year, expressed as daily height growth (lower part) and the accumulated height growth over the year (upper part). After Mitscherlich (1978).
During the life of a tree, height growth increases sharply after seedling establishment, reaches a maximum after 5-10 years and then slowly decreases again (fig. 14-3 and 14-5). The peak in height growth is referred to as the height growth culmination point. The cumulative height curve is the result of the integrated annual height growth over the entire age of the tree. The cumulative height curve shows an S-shape, where its inflection point corresponds to the culmination point of annual height growth curve. The culmination point of height growth is largely species-dependent and, within the same species, is achieved earlier on fertile sites (fig. 14-3).
For conifer species with monocormic crowns, the annual height increment can be derived from the internodes between the annual branch whorls (fig. 14-10). At higher age, tree height tends to reach a maximum, mainly determined by tree species and by site conditions (see 13.4). When a tree has reached its maximum height, height growth itself may not have stopped. In such old trees a top shoot may form every year, but the top regularly dies off after which height growth may be taken over by a side branch.
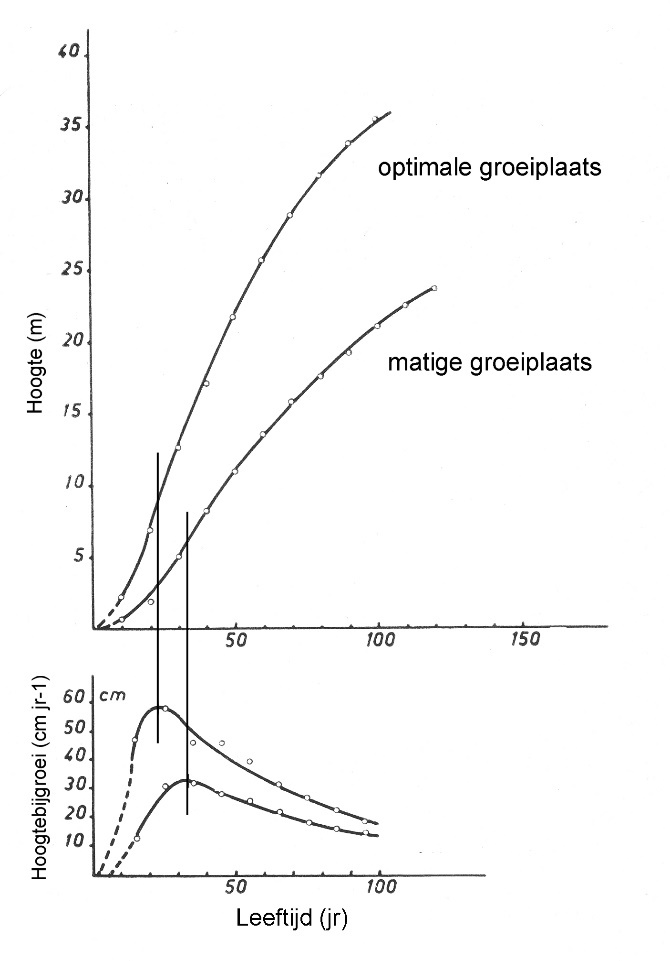
Figure 14-3: Height growth of Norway spruce at an optimal and moderate site. The culmination point (maximum) of the height growth curve (lower pane) corresponds to the inflection point in the height curve (upper pane), and is more likely to occur earlier in trees at the better sites (here around 23 years) compared to trees at moderate sites (here around 34 years). After Assmann (1961).
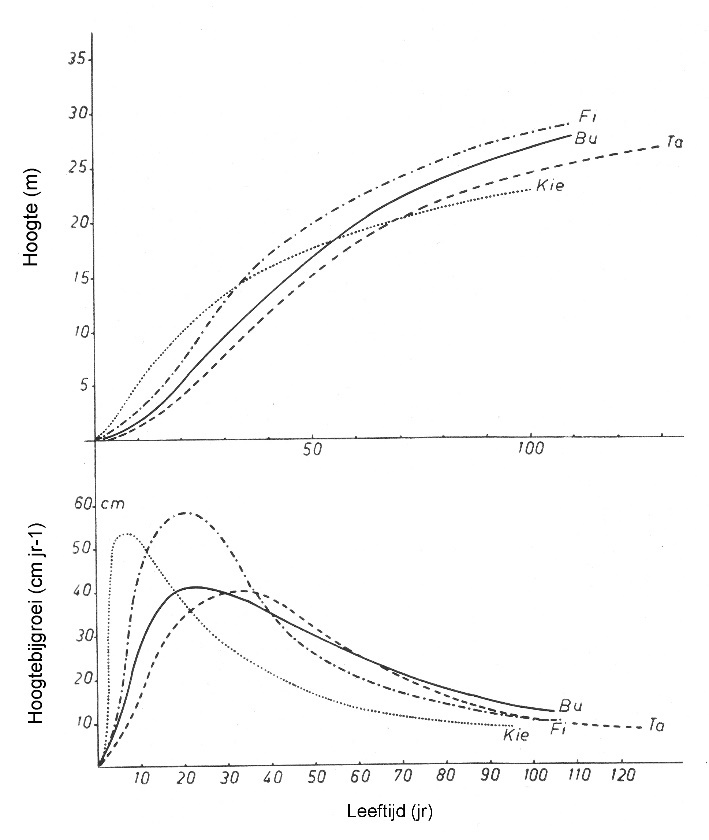
Figure 14-4: Height and height growth curves for four tree species at the same site. After Assmann (1961).
The differences in height growth result in rapid differentiation between different species within mixed stands (Fig. 14-4) and between individuals of the same species within monocultures (see 16.3.3). Species or individuals with rapid height growth in their first years are able to quickly acquire a favourable position in the canopy. For example, fast growing species such as larch, birch and alder may be one meter higher than potentially competing species within a few years, allowing these light-demanding species to grow in a mixture with slower-growing shade-tolerant species. When establishing mixtures of light-demanding species, it is important to choose species that have approximately an equal course of height growth (Jager & Oosterbaan 1994).
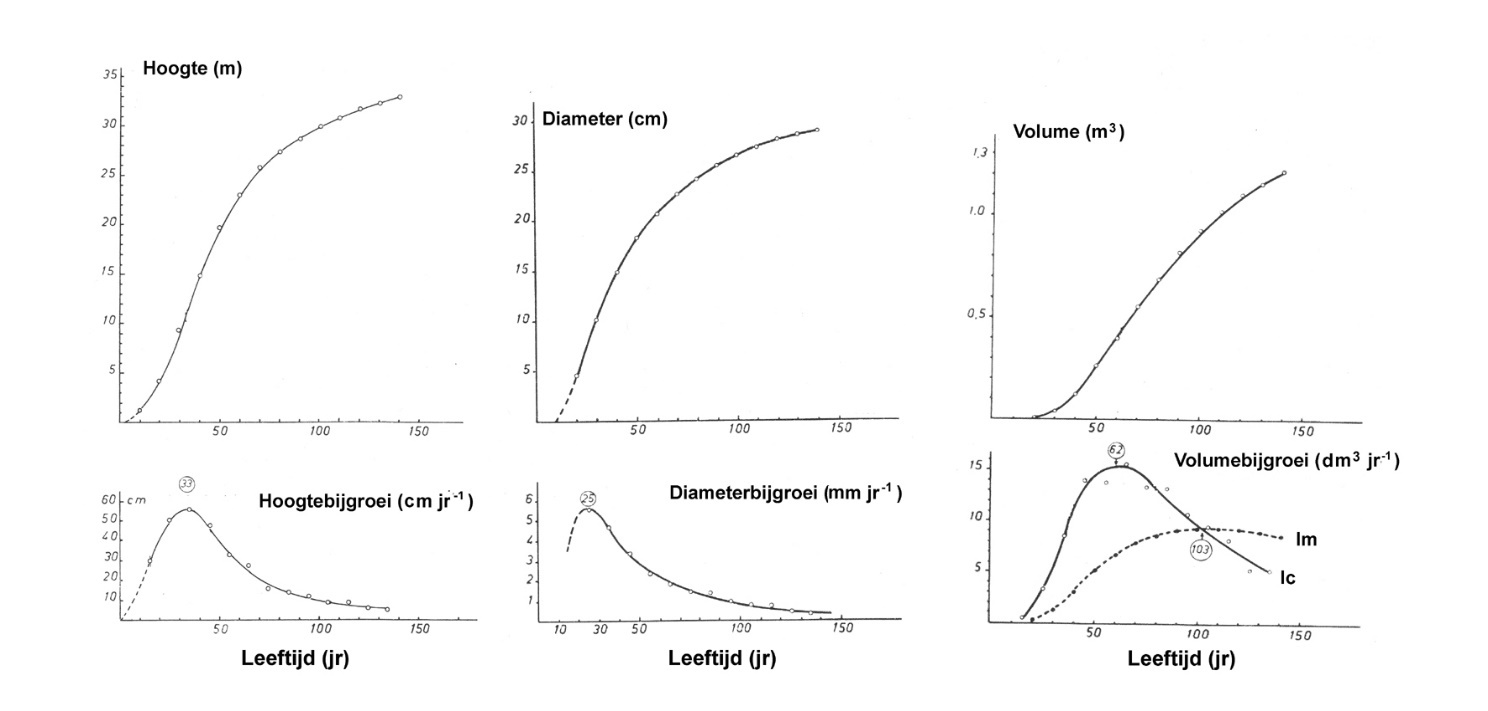
Figure 14-5: Evolution of the height, diameter and volume growth in time of a norway spruce (growth curves in lower panes, cumulative growth curves in upper panes). The numbers on top of the growth curves indicate the age of increment culmination. The volume increment (bottom right) is shown both as current periodic increment (Ic; see Table 14-1) and mean periodic increment (Im). After Assmann (1961).
Height growth in forest stands is usually measured by periodic recording of the height of the tree population (the stand). The most commonly used variables are the average height, the top height and dominant height (Table 14-1). In homogeneous, even-aged stands, the dominant height or the top height is hardly dependent on the density of the stand and is mainly determined by site quality. For this reason, the dominant height or top height of a stand at a certain age can be used as a measure of the growth potential (yield class or site class) of a species (fig. 16-16; also see 16.7.2). In very dense stands however, height growth may be lower due to intense competition for water and nutrients between individuals. Also in very open stands, the height growth may decrease because the individual tree growth is distributed over end meristems of a larger crown mantle that receives direct light (fig. 14-6).
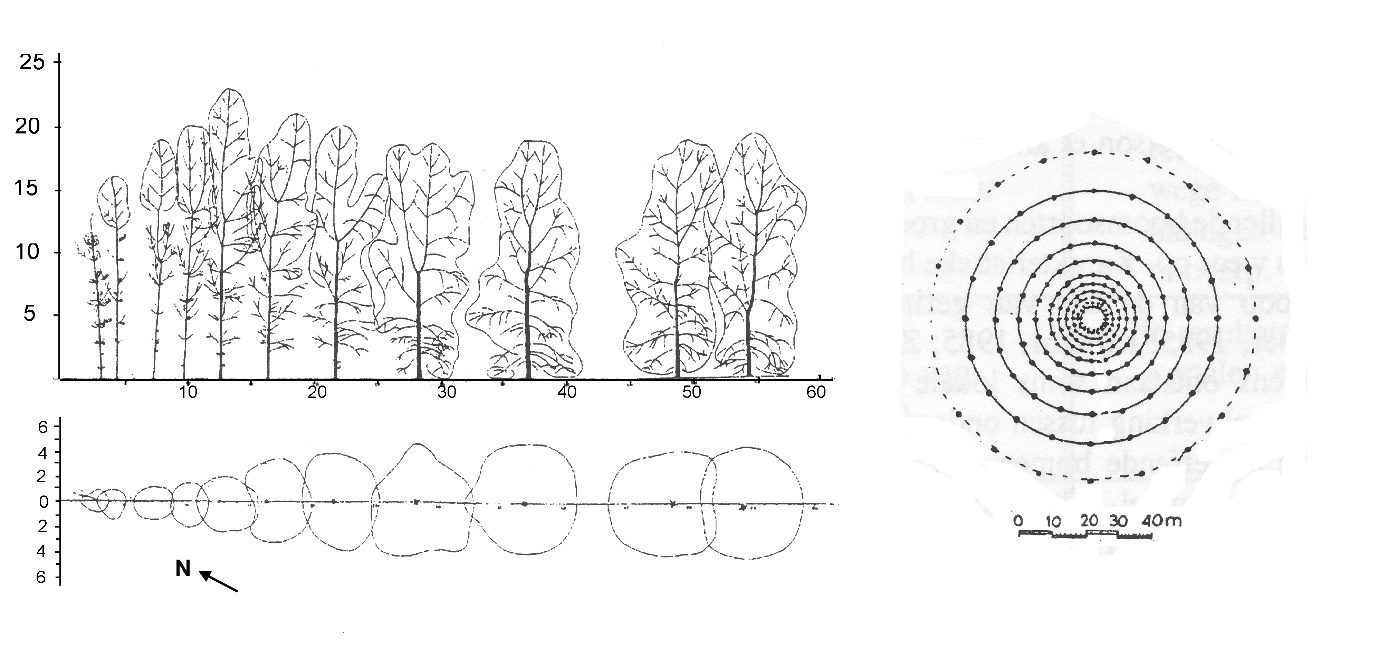
Figure 14-6: Transect drawing from a Nelder experiment with 14-year-old poplars (Populus x interamericana ‘Rap’) growing up in different densities. Side view (above) and crown projections (below) along one of the radii of the experimental design (right). From inside to outside the circle, the available growing space per tree increases gradually. After Houtzagers & Schmidt (1994)
Table 14-1: Common variables characterising tree dimensions and tree growth, and their aggregate values at the stand level. See also fig. 14-7. After Jansen et al. (1996).
| Variable | Level | Symbol | Unit | Definition |
| Height | tree | H | m | Vertical distance between the tree base and the tree top |
| Height increment | tree / stand | ih | m yr-1 | Height growth of individual trees |
| Length | tree | L | m | Distance between tree base and tree top |
| Dominant height | stand | hdom | m | Average height of the 100 tallest trees in a stand |
| Top height | stand | htop | m | Average height of the 100 largest trees in a stand |
| Average height | stand | hav | m | Average height of all canopy trees in a stand |
| Diameter at breast height | tree | Dbh | cm | Diameter of the stem at breast height (usually 1.30 m). |
| Diameter increment | tree / stand | idbh | cm yr-1 | Annual increase in diameter of the tree or average increase of all trees in the stand |
| Circumference | tree | C | cm | Circumference of the stem at 1.30 m or 1.50 m (in BE and FR). |
| Basal area | tree | B | m2 | Area of the stem cross section at dbh (1.30 m) |
| Basal area | stand | B | m2 ha-1 | Total area of all stem cross sections per hectare |
| Basal area increment | stand | IG | m2 ha-1 yr-1 | Annual increase of stand basal area |
| Quadratic mean diameter | stand | db | cm | Diameter at breast height of tree with average basal area |
| Stem volume | tree / stand | v V | m3 m3 ha-1 | Tree volume of the central stem from the base to the top |
| Merchantable stem volume | tree / stand | v V | m3 m3 ha-1 | Tree volume that can be used in processing the wood |
| Current annual volume increment | tree / stand | ic Ic | m3 yr-1 m3 ha-1 yr-1 | The increase in volume of the current tree or stand |
| Mean annual volume increment | tree / stand | im Im | m3 jr-1 m3 ha-1 yr-1 | The average increase in volume over the total life of the tree or stand. |
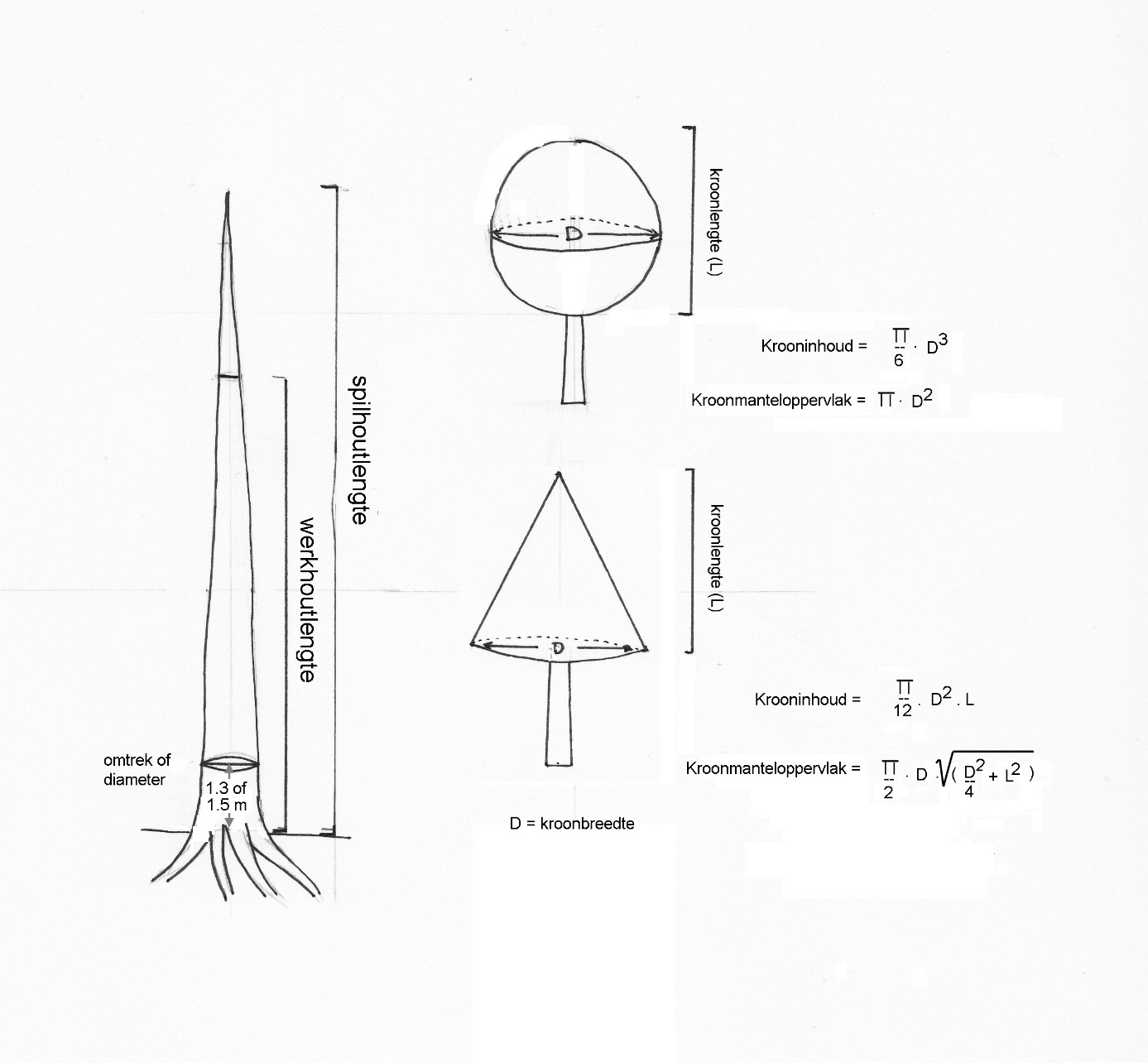
Figure 14-7: Some commonly used measures for determining tree dimensions with geometrical crown shapes.
14.3 Diameter increment
Diameter increment is the result of cambial activity in which new xylem and bark tissues are formed. The seasonality of xylem growth in temperate and boreal regions leads to the formation of annual rings, in which earlywood is formed in spring and latewood in summer (see 12.3.3). After an initial increase, during the juvenile growth phase of the tree, the diameter growth gradually decreases again. As with height growth, this leads to a peak in annual diameter growth (fig. 14-5), but this culmination point is usually reached later compared to height growth. As stand density (crowding) and degree of shading increase, the culmination point in diameter growth shifts to higher age. In practice, there is a large variation in diameter growth for a given age, resulting from the variation in environmental factors. When a tree grows up in the shade and is later exposed to full sunlight, the diameter growth may increase sharply at a later age. It is also possible that there are several periods with higher diameter growth, causing multiple peaks in the diameter growth curve. This is the case, for example, when a subcanopy tree undergoes several periods of light exposure as a result of thinning or death of surrounding trees (see fig. 25-17 – image of cross-section of a typical selection forest tree).
There are several reasons why diameter growth normally decreases with tree age. First, when volume growth remains the same, the xylem produced has to be distributed over an increasingly longer and thicker stem, and each layer of xylem hence tends to get thinner. Second, a tree maintains a functional balance between the water transport tissue (the xylem) and the foliage biomass under given site conditions. For the same transport capacity, a thinner ring of xylem is required with increasing diameter, while the same surface area of conducting tissue is maintained. In most species the central heartwood is no longer functional and there are no more respiration costs associated with it.
Diameter growth is mainly dependent on the canopy space available to a tree and the amount of sunlight intercepted by the foliage and is therefore primarily dependent on the stand density (see table 4-2 and fig. 4-8). With high stem density, and hence limited available growth space per tree, diameter growth is significantly lower than in stands of the same age with a lower stem number. Therefore, site productivity cannot be directly deduced from tree size, and thinning to reduce stem density is a very effective means to stimulate diameter growth (Savill & Sandels 1983; Balleux & Ponette 2006).
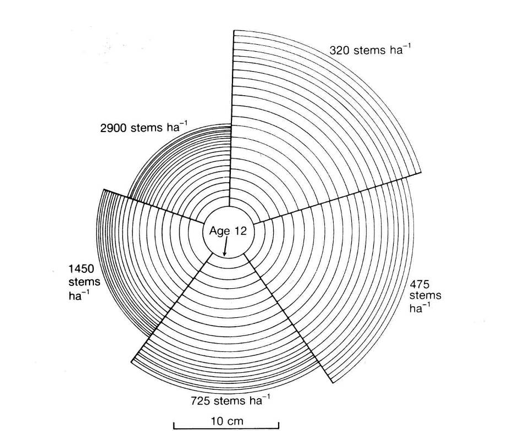
Figure 14-8: The effect of variation in growth space on the diameter growth at breast height (1.3 m) of Sitka spruce in Great Britain. The trees have grown up to an age of 12 y in the same density (2900 ha-1). After that, several densities were made by thinning once, and the trees were felled at the age of 32. To Savill & Sandels 1983.
Table 14-2: Influence of the planting density (square planting) on tree characteristics and stand growth in unthinned Pinus ponderosa in North America. The stand was measured in 1939, 1945, 1950 and 1959 and was 17 years old at the start of the measurements (Stiell 1966).
| Attribute | Unit | Spacing (m) | ||||
| 1.5 x 1.5 | 2.1 x 2.1 | 3 x 3 | 4.2 x 4.2 | |||
| Space per tree | m2 | 2.3 | 4.4 | 9.0 | 17.7 | |
| Stem density (n) | ha-1 | 4444 | 2267 | 1111 | 567 | |
| Top height 1939 | m | 7.0 | 6.8 | 6.1 | 6.0 | |
| Average height 1959 | m | 15.0 | 16.4 | 16.0 | 15.7 | |
| Average diameter 1959 | cm | 13.8 | 18.4 | 23.8 | 30.1 | |
| Crown length 1959 | m | 5.0 | 6.0 | 7.6 | 8.7 | |
| Crown width 1959 | m | 2.3 | 3.0 | 4.4 | 5.3 | |
| Branch diameter at crown base 1959 | cm | 1.8 | 2.2 | 2.9 | 3.7 | |
| Dry weight needles per crown 1959 | 103 kg ha-1 | 5.5 | 9.9 | 24.6 | 34.0 | |
| Dry weight needles 1939 | 103 kg ha-1 | 20.8 | 13.0 | 6.4 | 3.3 | |
| Dry weight needles 1959 | 103 kg ha-1 | 19.6 | 18.4 | 22.4 | 19.1 | |
| Stem increment 1939-45 | m3 ha-1yr-1 | 14.5 | 14.7 | 10.0 | 5.9 | |
| Stem increment 1945-50 | m3 ha-1yr-1 | 16.3 | 15.9 | 13.3 | 9.0 | |
| Stem increment 1950-59 | m3 ha-1yr-1 | 16.4 | 14.9 | 15.0 | 12.8 | |
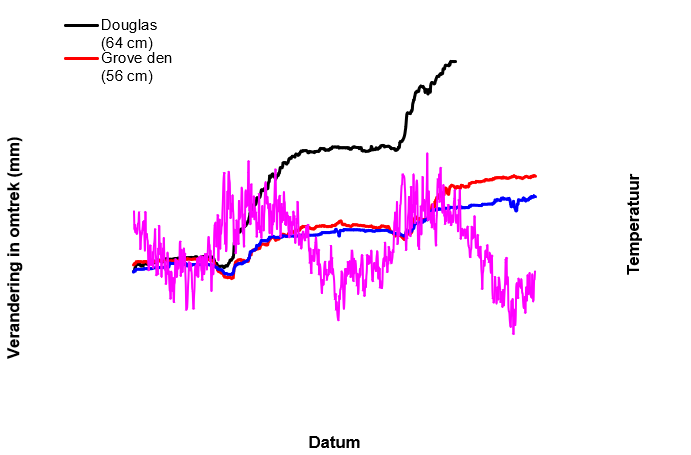
Figure 14-9 Tree circumference of a douglas fir, a Scots pine and a birch during two growing seasons, with corresponding daily temperature. Notice the sawtooth pattern of the cumulative curves, indicating shrinkage and swelling of the stem caused by variation in water content. Data from G.J. Nabuurs and E. Verkaik (WUR, Wageningen) measured in three Dutch forest reserves.
The stem diameter shows clear diurnal variation during the growing season. This variation is caused by variation in water content, caused by changing water tension within the xylem. During the day, at a high water transport and high tension, the stem may shrink slightly, while the stem recovers again when during nighttime transpiration stops and the water reserves in the stem are replenished (fig. 14-6). Note also that at the time of budburst in spring stem diameter decreases (fig. 14-9). It is not clear what causes this temporary shrinking. The radial (diameter) growth in a year is determined by the length of the growing season, more specifically by the number of growth days during the growing season in of temperate trees Etzold et al. 2022 (Ecol Letters).
Diameter growth is not the same along the axis of the stem. In a tree, smaller annual rings are formed at the base of the stem compared to higher positions along the stem (fig. 14-10). Over a year, diameter increase is largest in the lower part of the living crown where the amount of available assimilates is highest, and diameter increase is smallest at the base of the trunk. This differentiation in diameter growth along the stem ensures that the central stem does not develop like a cone, but that a very gradually tapered shape resembling a paraboloid is created, a shape between a cone and a cylinder. Tree species may vary in stem shape, and the extent to which the stem diameter decreases with height is referred to as taper (see Box 14.2 on bole shape and stem volume).
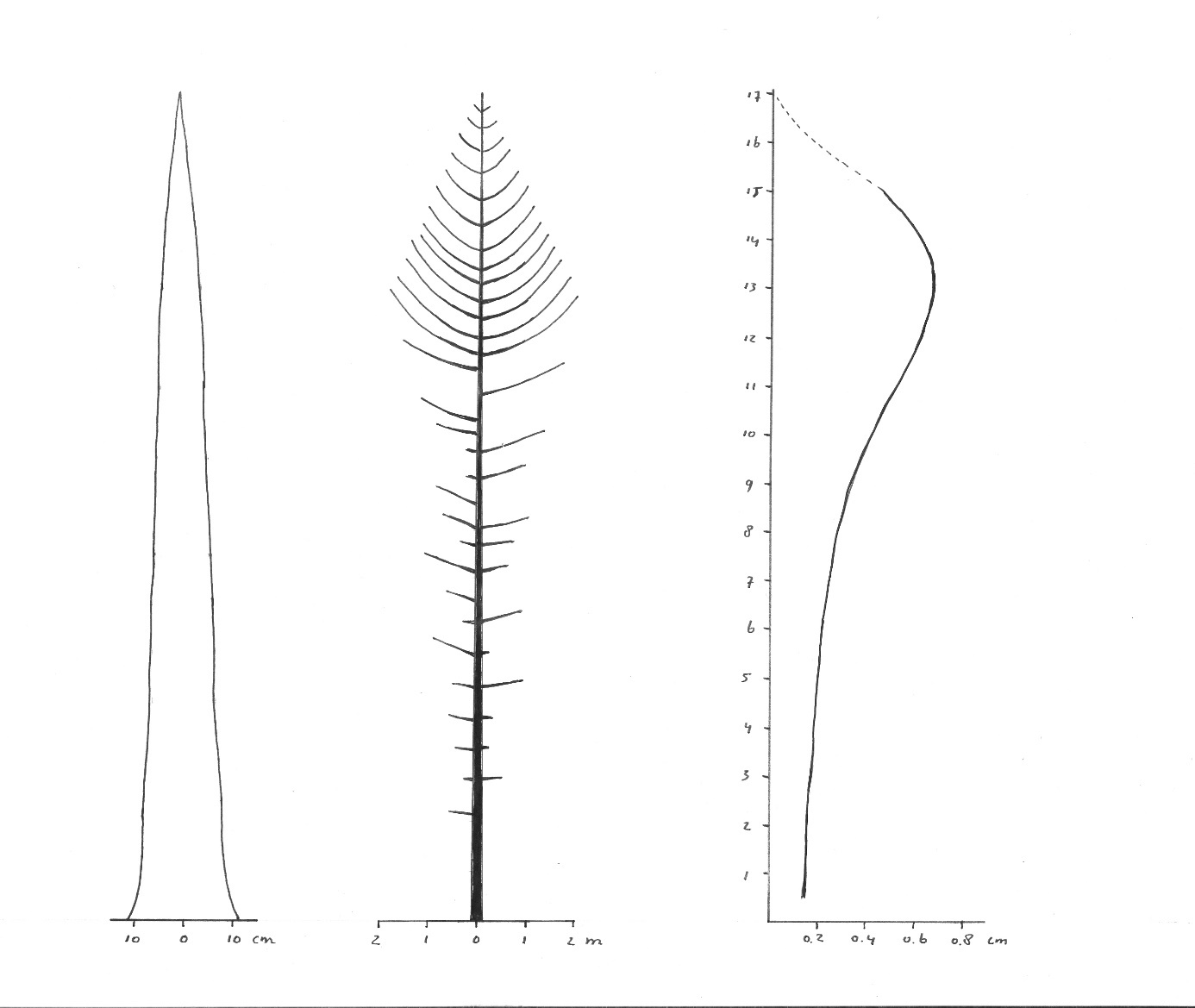
Figure 14-10: Stem shape (left), habitus (middle) and the relationship between tree height and the average annual diameter increase over the last five years (right) in a 38-year-old dominant Scots pine in an unthinned stand (unpublished data, Wageningen University).
Diameter growth can be measured by regular remeasurement of stem diameters at a reference height. The stem diameter of a tree is measured at a standard height from the stem base, the diameter at breast height, in most countries taken as 1.3 m above the forest floor. Exceptions are, amongst others, Belgium and France, where according to tradition stem circumference is measured at a height of 1.5 m. Repeated measurements in permanent plots are typically done every five or ten years.
14.4 Volume increment
The annual increase in height and diameter leads to an increase in stem volume. This volume growth is referred to as increment, and expressed in m3yr-1. The increment of an individual tree usually culminates at a later age than the diameter increase (fig. 1-5). The increment is usually not considered at the tree level, but rather at the stand level. The Current Annual Increment (CAI) is the actual increase in m3ha-1yr-1. In the case of permanent plots, this is often expressed as Periodic Annual Increment (PAI) in m3ha-1yr-1 calculated as the volume increase over the most recent observation period (e.g. the last five years). The average annual increment (Iav or Im, from Mean Annual Increment – MAI), is the increment calculated over the total stand age up to date, including stem volume possibly removed by thinning (fig. 14-11). CAI, PAI and MAI are concepts derived for even-aged stands, but can be developed for uneven-aged forests as well.
The growth of a tree’s stem volume depends on the amount of assimilates available for the production of new xylem in the stem. This depends primarily on the amount of photosynthesis, the respiration costs of maintaining the living tree biomass, and the way in which net growth is allocated over leaves, branches, stem and roots. Biomass increment declines when trees age, and there are two main factors that explain why also stand level increment decreases at higher stand age. First, in older stands the degree of crown closure will decrease, reducing light interception, and thus reducing photosynthesis per unit ground area. Furthermore, older stands reach their maximum height and resistance to transporting water is greater than with smaller trees, resulting in larger water shortage in the canopy in the case of drought. Water shortage in the crown leads to stomatal closure and reduces CO2 uptake for photosynthesis.
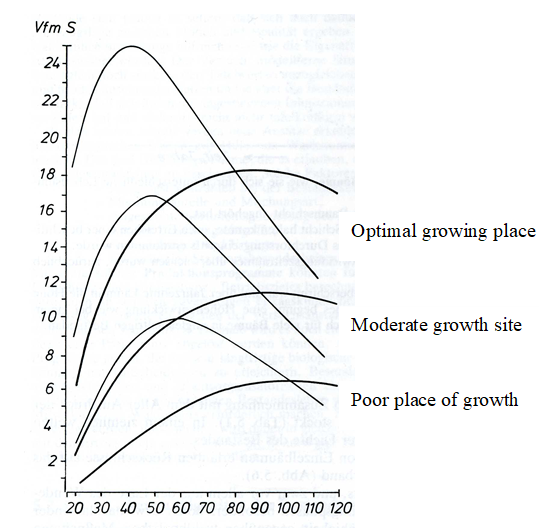
Figure 14-11: Evolution of current and mean annual increment at good, moderate and poor growth sites. Occurrence of maximum current and mean annual growth shifts towards higher ages with lower site quality. After Assmann & Franz (1963).
Theoretically, the age at which mean annual increment (Im) culminates in an even-aged stand, indicates the rotation length with the highest possible stem volume production for a given tree species at a given site. The use of this production indicator leads to relatively short rotations, and is in principle well suited for maximum volume or biomass production. This usually concerns low quality applications such as firewood or fibre wood (for paper or fibre board applications). However, when larger dimensions and higher wood quality is desired, rotation length has to be increased and total biomass production is less relevant and mass production is replaced by quality production. Only at higher age stems develop knot-free logs, with a sufficiently thick layer of knot-free wood for use for veneer or furniture. Large dimension sawn timber also requires larger stem dimensions, with higher prices per unit volume for older and larger stems. Thus despite lower average growth over a longer rotation, higher financial yield can be achieved. This means that the culmination of the value gain (in € yr-1 for a tree or in € ha-1yr-1 for a stand) usually occurs at a later age (fig. 14-12). Also natural forest regeneration will require longer rotations allowing time for sexual reproduction.
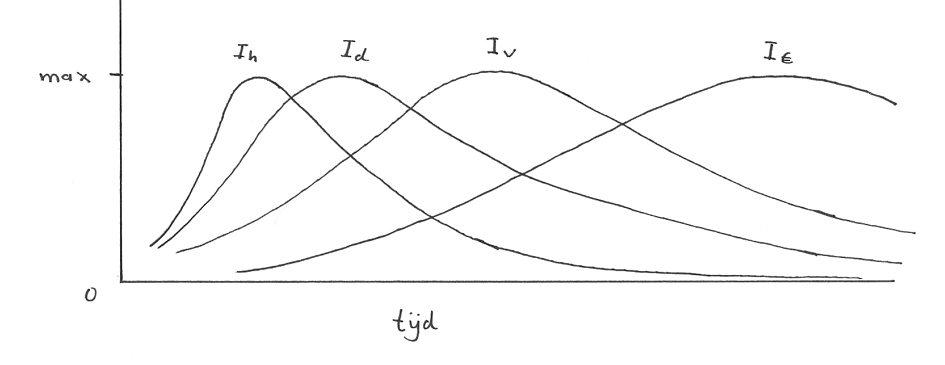
Figure 14-12: Generalized curves of the annual increment of height (Ih), diameter (Id), volume (Iv) and value per m3 (I€) of a tree. The culmination of increment falls at different times for the different variables.
As a step in the calculation of the volume of trees and stands, and for determining their increment, the basal area is used. Basal area is defined as the cross-sectional area of a stem or of a stand at breast height (1.30 m). For an individual tree, it can be derived directly from the measured diameter (dbh) using:
b = ϖ ∙ (dbh / 2)2
In the case of a measured circumference (c), the basal area is equal to:
b = c2 / (4 ∙ ϖ)
It is custom to express the basal area of a stand as the sum of all individual tree basal areas per hectare. This basal area (B) is a commonly used measure of stand density, as it combines tree density and tree size. Stand basal area tends to reach a maximum value in dense stands. The need for, or intensity of, thinning can be derived from comparison with guidelines for basal area derived from permanent plots. For shade-tolerant tree species, a higher basal area can be maintained compared to light-demanding species (see 3.4.2).
Various measurement protocols have been developed to determine the volume of a stand (see classical text books such as Prodan 1965, Loetsch & Haller 1971, or de Vries 1986 FM: needs updating). Essentially, the volume of a stand can be estimated by multiplying stand basal area with average height, and correcting for tree shape using an overall form factor reflecting the taper of the trees (see Box 14-2 on tree shape and stand volume). The form factor in general lies between 0.40 and 0.45, reflecting a general bole shape between a cone (form factor 1/3 = 0.33) and a paraboloid (form factor ½ = 0.5). The average height of the stand can be approximated by taking the height of the tree with the quadratic mean diameter (db), i.e. the model tree with the average basal area in the stand (Table 14-1). Although the height of the tree with quadratic mean diameter is slightly more than the actual average height, it can be used to estimate the volume of the stand (Prodan 1965). If the volume of the tree with quadratic mean diameter (v) is known, stand volume (V in m3ha-1) can be estimated by multiplying the volume of the tree with mean quadratic diameter by the stem number (N) using V ≈ N ∙ v
A more accurate, but also more intensive, method is based on the actual tree population, based on circular sample plots circles, or using a complete tally in which diameter and height of all trees in the stand are determined, and the shape factor or other volume function per individual tree or per diameter class is applied. The literature on methods of forest inventory and wood geometry is large. For more detailed considerations, see overview books such as Philip (1994), Van Laar & Akça (2007) and West (2009) (FM: needs updating).
16.5 Root growth
Roots, as in the case of above-ground stems and branches, grow in length and thickness. Roots can be separated in fine roots and coarse roots. The fine roots (with a diameter of less than 2 mm) grow mainly in length, and growth in root diameter is mainly concentrated in coarse roots. Fine roots have a lifespan of about a year, and are often formed continuously when conditions for root growth are favourable. For the absorption of water and nutrients, the fine roots exploit a large part of the soil volume, as movement of water and nutrients in soil tends to be slow. To exploit the soil volume, up to thousands of km of fine roots can be present in the soil per hectare, with a majority of roots not going deeper than 1 m (Table 14-3). The coarse roots mainly serve for the transport of water and nutrients, and they also play an important role in anchoring the tree in the soil. Ultimately, the belowground root volume is significantly less than the volume of the above-ground parts (Table 14-3). In temperate climate zones, the root/shoot volume ratio is about 1/5, but in drier zones, trees invest relatively more in roots.
The root growth, notably root extension, can be very dynamic because root hairs appear and disappear almost continuously (fig. 14-13). Like the above-ground parts, root growth during the growing season is mainly influenced by temperature and moisture availability. During a long dry period, many fine roots may die, and new fine roots are formed again when soil moisture increases. The periodicity of root growth during the growing season may vary significantly from the above-ground biomass, as roots exhibit a longer growth period during the year (fig. 14-13). First of all because roots respond to different hormonal signals compared to the above-ground biomass, resulting in the onset of growth well before the above-ground biomass starts to grow. This is necessary to provide the above ground parts with sufficient water and nutrients during the bud flush (but see fig. 14-13 for different patterns per species). While onset of root growth can be earlier, it also may continue longer in the season because of the buffered soil climate, where a decrease in air temperature only gradually leads to a decrease in soil temperature.
Table 14-3: Root characteristics of three types of forest stands on a moraine soil in Denmark. Source: Holstener-Jørgensen (1958).
| Attribute | Pedunculate oak (48 yrs) | European beech (63 yrs) | Norway spruce (44 yrs) | ||
| Root length (km) | 739 | 2520 | 1330 | ||
| Root weight (ton) | 10.6 | 21.5 | 31.8 | ||
| Root volume (m3) | 17.4 | 39.1 | 51.3 | ||
| Trunk (m3) | 20.0 | 37.8 | 32.1 | ||
| Total below ground volume (m3) | 37.4 | 76.9 | 83.4 | ||
| Above ground volume (m3) | 201 | 316 | 332 | ||
| Percentage of roots (% of m3) | 18.6 | 24.3 | 25.1 | ||
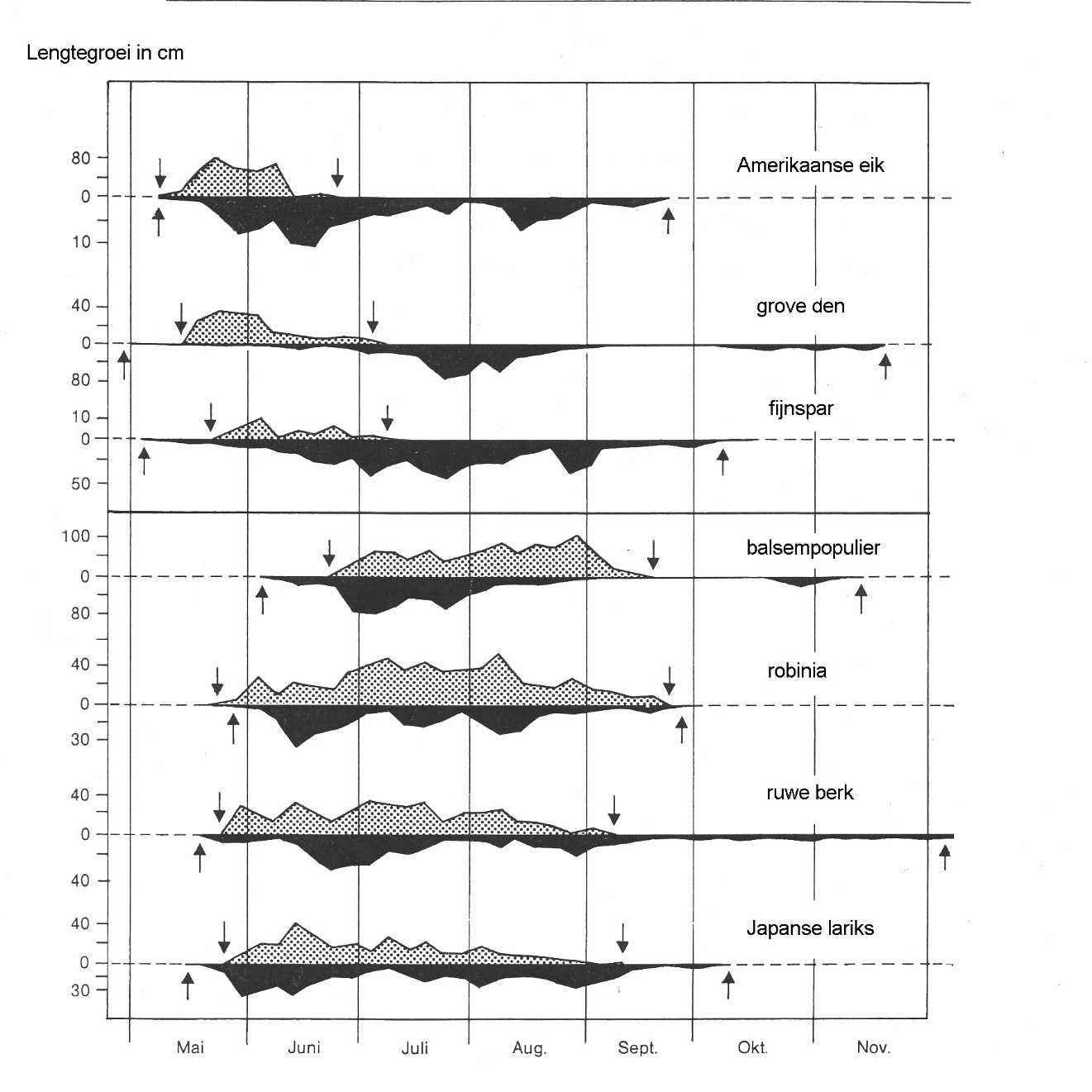
Figure 14-13: Variation in the elongation of the top shoot (above zero line) and of the roots (below zero line) during the growing season for seven tree species in Eberswalde (D). The arrows indicate the beginning and end of the growth period. (Lyr et al. 1992).
14-6 Development stages
Based on the rhythm of height and diameter growth, the life cycle of a tree can be divided into three stages: the juvenile phase dominated by height growth, the adult phase dominated by diameter growth and with occurrence of flowering and seed production, and the senescent phase characterized by decrease in crown density and eventually deterioration of the entire crown (fig. 14-14). The speed at which these phases succeed each other is to a large extent species-specific and determines the harvest age that foresters use. Note that pioneer tree species with high light demands tend to have a short life span, while shade-tolerant late-successional species may reach much higher ages. Birch, willow, poplar and cherry trees have a relatively short life span not exceeding more than 100-150 years. Oak and lime on the other hand, may reach ages of several hundreds of years (Table 14-4).

Figure 14-14: Declining beech on a drift sand wall on the Veluwe area (Netherlands). During decline, the crown slowly degenerates and the leaves concentrate more and more around the crown base. Photo Jan den Ouden.
Table 14-4: Maximum diameter, height and age of a number of important tree species from the Netherlands and Flanders (FM: needs updating with Europe-wide data, see EUFORGEN Atlas). The average maximum age expectation is lower than the values given here. Higher maximum values have been found elsewhere in Europe. Data collected by L. Goudzwaard.
| Species | Maximum diameter (cm) | Maximum height (m) | Maximum age (yr) |
| Beech | 270 | 45 | 250 |
| Corsican pine | 140 | 45 | 500 |
| Common ash | 180 | 45 | 250 |
| Norway Spruce | 115 | 41 | 400 |
| Common maple | 165 | 38 | 300 |
| Scots pine | 100 | 40 | >500 |
| Hornbeam | 180 | 20 | 250 |
| Silver birch | 105 | 25 | 120 |
| White willow | 230 | 34 | 100 |
| Yew | 170 | 20 | >500 |
| Sessile oak | 155 | 33 | >500 |
| Small-leaved lime | 220 | 35 | 500 |
| Sweet cherry | 120 | 30 | 70 |
| Pedunculate oak | 245 | 41 | >500 |
| Black alder | 140 | 30 | 300 |
| Black poplar | 155 | 30 | 200 |
14.7 Variation in growth
Trees vary in growth rate, depending on their age, their genetic predisposition and the availability of growth factors such as light, water and nutrients. In the end, very large differences may arise in the final tree size. For example, on very dry and acidic soils, Scots pines do not grow much taller than 10-15 m, while on a rich soil with good moisture supply, the same species can easily reach 30 m in height. In the deep shade, a beech may not have grown much taller than a few meters over a period of a 100 years, while in full light, in the same time and at the same site, a beech tree may grow up to a height of about 40 meters.
The large variation in independent site factors for tree growth, such as climate and soil conditions, is clearly reflected in the differences in site productivity for a given tree species observed between stands, forests or regions (Table 4-5). Furthermore, tree species may have an effect on soil formation and soil organic matter, via their litter quality (see 11.2.2) and hence may have a strong influence on water availability and nutrient supply of the soil (Hommel et al. 2007). This also strongly influences the growth of trees, and the herbaceous flora under the canopy, and depending on the tree species, large differences in growth and tree vitality may occur at small spatial scale.
This section first discusses the relationship between the growth of individual trees and the growth of a stand, and subsequently growth differences between stands are discussed based on expected growth based on site quality. At the end, mathematical models to predict tree and stand growth are briefly introduced.
14.7.1 From tree increment to stand growth
For several reasons, e.g. to determine the influence of different soil types on growth, it is important to translate individual tree increment into the growth of the entire stand. Usually, it is not possible to accurately estimate the specific growing conditions of an individual tree in a stand, e.g. because it cannot be determined in a non-destructive way where the roots are located. Also, light interception by individual crowns cannot be determined with simple measurements. At the level of a stand, however, it is possible to determine the total amount of available radiation, or the moisture availability in the rooted profile. Thus, when assessing growth in relation to site factors, the total growth per unit of area (per hectare) is a more suitable variable to consider.
The diameter growth of individual trees is not a good indicator of site quality. When stem number is high, diameter increment will be lower at certain stand growth compared to a stand with lower stem number. It is therefore more appropriate to consider basal area increment for the entire stand (the sum of tree basal area in m2ha-1). If, as a result of thinning, the diameter increment of the remaining trees increases, the stand growth may still be lower than before the thinning, e.g. because the total light interception has decreased due to thinning.
14.7.2 Yield class and increment prognosis
Given the long life span and growth period of trees, the prediction of growth in forestry is of great importance. In the past, much attention has been paid to the development of growth descriptions using empirical yield tables (Table 4-6). A yield table is an empirical model predicting the development of stand volume, tree height and other stand characteristics over age for a given tree species, usually in even-aged monocultures, separated per site class (see below). Such tables are based on empirical data from permanent field plots, and apply to stands of a particular tree species with the same thinning regime in a specific geographical region. Yield tables are used, among other things, for a quick assessment of increment, and for the prediction of yields from thinning and final felling. They also serve as a standard or guideline for thinning treatments. For the Netherlands, yield tables been compiled Jansen et al. (1996). Specifically for Flanders, only yield tables for Corsican pine have been developed (Meuleman et al. 1991). Dagnelie et al. (1988) have drawn up yield tables for norway spruce in Wallonia. (FM: needs updating and expanding; Herziene opbrengstabellen voor Vlaanderen (2020) hebben we met Hans Jansen zaliger gepubliceerd, zie https://ecopedia.s3.eu-central-1.amazonaws.com/pdfs/Opbrengsttabellen%20Vlaanderen%202020_final.pdf)
The growth potential of a tree species for a specific site is called yield class or referred to as site quality. The yield class can be expressed directly as the maximum average annual volume increment (in m3 ha-1 yr-1), or categorized as yield or site classes (Table 14-6, Fig. 14-15). Volume growth is strongly correlated with height growth. This is reflected in Eichhorn’s law (1904), which states that at a certain average stand height, the volume is the same regardless of the site quality of the stand. In later studies, it became clear that this was not valid under all conditions (Prodan 1965), but it clearly indicates that stand height (at a certain age) provides a good measure for estimating stand volume and productivity. The yield classes in the yield tables are therefore defined on the basis of dominant height, attained at a certain age (fig. 16-15).
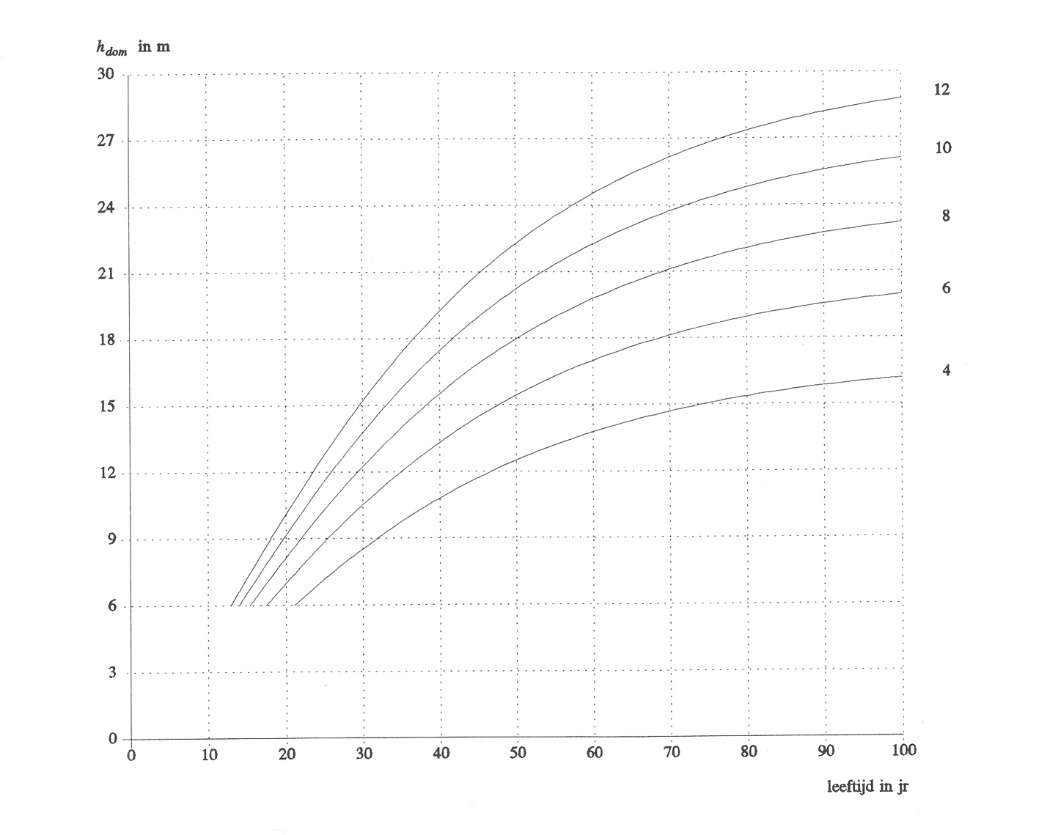
Figure 14-15: Height curves of Scots pine for different site classes in the Dutch yield table for Scots pine (Jansen et al. 1996). The site classes are expressed as the volume increment value at culmination point of the mean annual increment Im (in m3 ha-1 yr-1). By determining the dominant height of a stand of a known age, site class can be inferred from the graph.
Table 14-5: Relationship between site class, volume production, dominant height at age 100, and independent site factors for beech in Belgium.
| Site class | Ecoregion and soil | Hdom (m) | Increment (Im) (m3 ha-1 yr-1) |
| I | Brabant district (loam soil) | 35-40 | 8-10 |
| II | Flemish district (sandy loam soil) | 30-35 | 6-8 |
| III | Ardennes (shallow loam on schist rock) | 25-30 | 4-6 |
| IV | Kempen (sandy soil) | 20-25 | 2-4 |
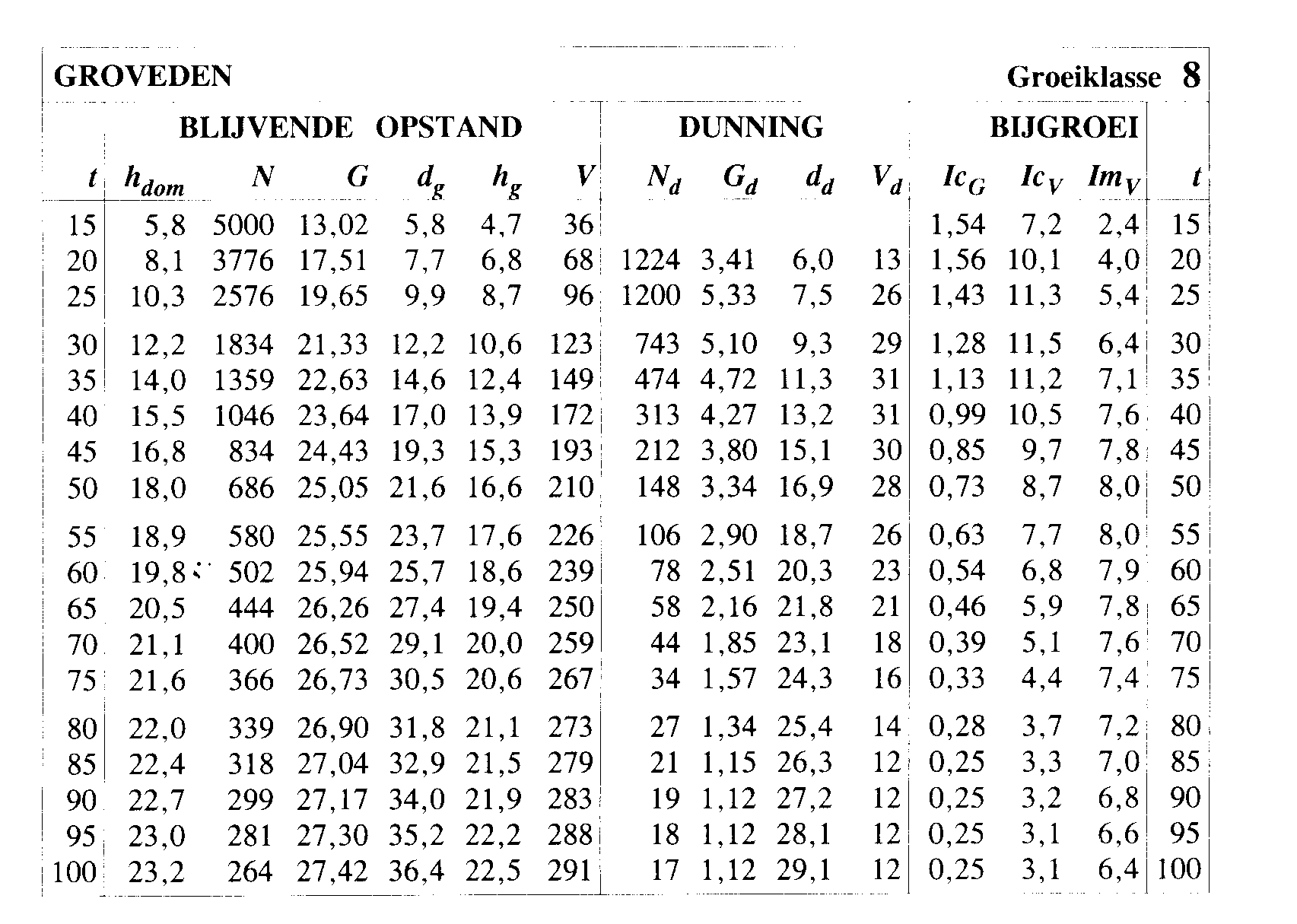
Table 4-6: Example of a yield table for Scots pine of site class 8, from Jansen et al. (1996, see also fig. 14-15), with t = age (yr), hdom = dominant height (m), N = stand density (ha-1), B = stand basal area (m2 ha-1), db = quadratic mean diameter (cm); hb = height of the tree with quadratic mean diameter (m); V = stand volume after thinning (m3 ha-1), Nth = number of stem in the thinning; Bth = basal area of thinning (m2 ha-1), dth = quadratic mean diameter of thinning (cm); Vth = volume of thinning (m3 ha-1), IcB = current basal area increment at age t (m2ha-1yr-1), IcV = current annual volume increment at age t (m3 ha-1 yr-1), ImV = mean annual volume increment (m3 ha-1yr-1).
In the development of yield tables, and also in other studies that model tree growth, generalized functions are used to describe the development of particular tree characteristics over time, based on measurements at permanent field plots, usually in even-aged stands. Increment curves can be of various mathematical forms, depending on the purpose and on the availability no data. Increment curves are derived by fitting the most appropriate mathematical formula to empirical tree growth data. One of the most commonly used expressions for estimating the volume growth of homogeneous even-aged stands in relation to site quality is the so-called Chapman-Richards equation:
Y = a · (1 – e-b t) c
with Y = the variable to be predicted, a = a maximum value that variable Y may reach (asymptote), b = parameter describing the growth rate, and c = a shape parameter for the expression and t = age of the tree or stand. The Chapman-Richards function has been widely used for modeling height growth, as height tends to reach a maximum at higher stand age (the asymptote a in the expression). The increase of basal area with age can also be described with the Chapman-Richards function.
An important limitation of the empirical yield tables is that they do not take into account any changes in growing conditions during the life of a stand. For example, Spiecker et al. (1996) have shown that the majority of older stands in Europe have increased in productivity during their life span, comparable to at least one site class, as a result of nitrogen and CO2 fertilization (Pretzsch et al 2024; Etzold et al 2020). Furthermore, yield tables are limited to the stand and site conditions of the field plots on which they are based. For example, the German or Dutch growth tables are not suitable for the beech stands in the Sonian Forest in Belgium. These beech stands show a significantly slower growth in their youth (during the first 40 years) due to the presence of a strongly compacted and decalcified loam layer in the upper soil layers. At a later age (around 120 years) the stands exhibit a stand increment that is higher than the best site class from the yield tables, as a result of the fact that their root system has expanded downwards and has reached the calcareous loess that is present at about 3 m depth (see De Vos, 2005).
In contrast to empirical growth models such as yield tables, mechanistic growth models explicitly take into account the influence of individual growth factors, making it possible to predict growth under changing conditions. Mechanistic models are based on the ecological and physiological processes underlying growth (photosynthesis, respiration, evapotranspiration, etc.) and may simulate the growth of a stand based on ecological processes (e.g. Mohren 1987; Deckmyn et al. 2008). The disadvantage of these models is their high degree of complexity and therefore high need for data on site conditions, and species-specific parameters characterizing physiological processes. In combination with the uncertainties in the quantification of the most important physiological processes, this leads to mechanistic models being particularly suitable for research on forest ecosystems by testing hypotheses through simulation experiments, and less for the actual prediction of growth in a concrete situation. Promising alleys to predict forest growth under climate change are hybrid models, which combine the predictive capacity of mechanistic models with the accuracy of empirical models (e.g. Storms et al. 2022)
Recommended literature: Assmann (1961), Mitscherlich (1978), Lyr et al. (1992), Pretzsch (2009).
Literature
Assmann, E. 1961. Forest yield science. FSVO, Munich.
Assmann, E. & F. Franz 1963. Preliminary spruce yield table for Bavaria. Institute of Yield Science of the Forstliche Forschungsanstalt München, Munich.
Balleux, P. & Q. Ponette 2006. Spruce thinning device, principles lessons from thirty years of experience. Walloon Forest 83: 3-21.
Berman, M. E. & T. M. De Jong. 1997. Diurnal patterns of stem extension growth in peach (Prunus persica): Temperature and fluctuations in water status determine growth rate. Physiologia Plantarum 100: 361-370.
Dagnelie, P., Palm, R., Rondeux, J., Thill, A., 1988. Production tables relating to common spice. Les Presses agronomiques de Gembloux.
Deckmyn, G., H. Verbeeck, M. Op de Beeck, D. Vansteenkiste, K. Steppe & R. Ceulemans 2008. ANAFORE: a stand-scale process-based forest model that includes wood tissue development and labile carbon storage in trees. Ecological Modeling 215: 345–368.
De Vos, B., 2005. Soil compaction and the influence on the natural rejuvenation of Beech in the Sonian Forest. Institute for Forestry and Wildlife Management, Report IBW Bb 2005.004.
De Vries, P.G. 1986. Sampling theory for forest inventory. A teach-yourself course. Springer Verlag, Berlijn.
Eichhorn, F. 1904. Relationships between stock level and inventory mass. Allgemeine Forst und Jagdzeitung 80: 45–49.
Holstener-Jørgensen, H. 1958. Investigation on root systems of oak, beech, and Norway spruce on groundwater affected moraine soils with a contribution to elucidation of evapotranspiration of stands. Forstlige Forsögsväsen I Danmark, Bd. 25, 225–290.
Houtzagers, M.R. & P. Schmidt 1994. The reaction of poplar clones to competition in relation to growth space. Hinkeloord Reports 12, Wageningen Agricultural University.
Jager, K. & A. Oosterbaan 1994. Construction of mixed hardwood plantings with native species. Schuyt & Co, Haarlem, The Netherlands.
Jansen, J.J., J. Sevenster & P.J. Faber 1996. Yield tables for important tree species in the Netherlands. IBN report 221 / Hinkeloord Report 17. IBN-DLO / Agricultural University Wageningen, Wageningen.
Leibundgut, H, S. Dafis & F. Richard 1963. Studies on root growth of different tree species. Schweizerische Zeitschrift für Forstwesen 114: 621-646.
Loetsch, F. & K.E. Haller 1973. Forest Inventory (2 delen). FSVO, Munich.
Lyr, H., H.J. Fiedler & W. Tranquilini 1992. Physiologie und Ökologie der Gehölze. Gustav Fisher Verlag, Jena.
Meuleman, B., F. Dufrane, & G. Carletti 1991. Production table for Corsican pine in Flanders. LISEC, Genk, The Netherlands.
*Mitscherlich, G. 1978. Wald, Wachstum und Umwelt. Volume I: Form and Growth of Baumund Bestand. Frankfurt a.M., J.D. Sauerländers Verlag.
Mohren, G.M.J. 1987. Simulation of forest growth applied to douglas fir stands in the Netherlands. Proefschrift, Landbouwuniversiteit Wageningen, Wageningen.
Philip, M.S. 1994. Measuring Trees and Forests. Wallingford, CABI Publishing, 2nd edition..
Pretzsch, H. 2009. Forest Dynamics, Growth and Yield. Berlin, Springer Verlag.
Pretzsch et al. 2014. Forest stand growth dynamics in Central Europe have accelerated since 1870. Nature Communications (check)
Etzold et al. 2020. Nitrogen deposition is the most important environmental driver of growth of pure, even-aged and managed European Forests. Forest Ecology and Management (check)
Prodan, M., 1965. Holzmesslehre. J.D. Sauerländer’s Verlag, Frankfurt am Main.
Savill, P.S. & A.J. Sandels 1983 The influence of early respacing on the wood density of Sitka spruce. Forestry 56: 109-120.
Spiecker, H. K. Mielikäinen, M. Köhl, J.P. Skovsgaard (eds.) 1996. Growth Trends in European Forests. Studies from 12 Countries. European Forest Institute Research Report No. 5, Springer Verlag, Berlin..
Stiell, W.M. 1966. Red pine crown development in relation to spacing. Department of Forestry Publication 1145, Ottawa.
Storms, I., Verdonck, S., Verbist, B., Willems, P., De Geest, P., Gutsch, M., Cools, N., De Vos, B., Mahnken, M., Lopez, J. and Van Orshoven, J., 2022. Quantifying climate change effects on future forest biomass availability using yield tables improved by mechanistic scaling. Science of the total environment, 833: 155-189.
Van Laar, A. & A. Akça 2007. Forest Mensuration. Springer, Dordrecht, the Netherlands.
West, P.W. 2009. Tree and Forest Measurement. Springer Verlag, 2nd edition, Berlin.
Box 4-2: Tree shape and stem volume
The shape of a tree stem determines the total volume, strength properties, and quality of the sawtimber. The shape also partly determines a number of physiological processes such as water transport. The volume of a tree stem is usually calculated from the stem length or tree height (h, in m), and the thickness or diameter of the stem at some reference height (dbh, in cm). A stem is best measured after a tree has been felled, for example by section measurements in which the length and diameter of individual stem segments are measured at the lower and at the high end. If the stem shape is equal to a cylinder, the volume (v, in m3) can be simply calculated as: v = (dbh/200)2 h However, the diameter decreases from the stem base to the top of the tree.
To accurately determine stem volume, felled trees can be divided into sections of 1-2 m, the volume of which is then measured separately and the entire stem volume determined by addition. For each section, it is assumed that the diameter decreases proportionally with the length of the stem part (section), and that the section has the shape of a truncated cone. The volume of such a stem section i can then be estimated according to Huber’s method as vi = bmi Li, where bmi is the mean basal area in m2 of the i-th stem section, calculated as ((dbh1+dbh2)/400)2) in which dbh1 and dbh2 are the diameters of the section ends, and Li is the length of the stem section. If the shape of a stem section deviates strongly from a cylinder, it is desirable to measure many sections to make the measurement error as small as possible.
For standing trees, stem volume is estimated from dbh and total tree height. Diameter at breast height is assumed equal to the diameter at the stem base, and the volume must be corrected for the deviation of the stem shape from a cylinder with diameter dbh and height L. This correction factor is called the tree form factor when determining the volume of an individual tree. When the stand volume is estimated, an average shape factor for all trees is used: the stand form factor. The form factor equals the ratio between the actual tree volume and the volume of a cylinder of equal diameter and length. When a tree has a high form factor, and the diameter of the trunk only slowly decreases with increasing height, the stem has a small gradient and it is called solid.
The shape of the tree stem can be approximated with a mathematical rotational body such as a paraboloid or a cone, so that a fixed form factor f can be used to determine the stem volume in relation to the volume of a cylinder of equal diameter and length (see fig. B1). In the formula v = f (dbh/200)2 L, the values for f for a cylinder, a paraboloid, a cone and a neiloid are 1, 1/2, 1/3, and 1/4 respectively (the factor for a neiloid depends on the curvature and can be between 0 and 1/3). The shape of a tree stem depends on the species and the place of growth, but is often somewhere between the value for a cone (1/3) and a paraboloid (1/2). For a rough estimate in the field, a value between 0.4 and 0.45 can be used for f.
A: f = 1 B: f = 0.5 C: f = 0.33 (D: f = 0.25)
v = f (dbh/200)2 L
Figure B4-2-1: Theoretical tree shapes with the corresponding form factors for calculating the tree volume. A=cylinder, B=paraboloid, C=cone and D=neiloid
In yield tables, a volume function based on a regression equation is often used, calibrated on section measurements of a sufficiently large number of felled logs. A commonly used volume function is v = a dbh b L c, which is a more general form of the expression : v = f (dbh/200)2 L. This relationship between dbh, h and v can then given in so-called volume tables. For forest inventory, a relationship between the diameter and the tree volume can then be derived by measuring a number of test trees in an area via the volume table. Through regression analysis, a relationship can then be established between dbh and v, usually using a second-degree polynomial. The resulting volume tariff then takes the form: v = a + b dbh + c dbh2.
BOX 14-1 Lammas shoots
Lammas shoots develop when the newly formed buds of the spring shoot sprout during the same growing season (Figure B4-1-1). In some regions these extra shoots are called Saint John’s shoots as in the case of oak this second flush used to appear around June 24, the day of St. John. Under the current, warmer climate conditions, flushing already falls in the early June. In favourable weather conditions, a third, and sometimes even a fourth shoot may occur during the course of summer. In douglas fir, Lammas shoots only occur in late summer.


Figure B4-1-1: Lammas shoots on pedunculate oak (left) and sweet chestnut (right). Photos Jan den Ouden.
A majority of the deciduous and coniferous species develop lammas shoots. In pedunculate oak, chances of lammas shoots occurring decreases with the size of the tree. Mature trees only make lammas shoots incidentally in their crown, although in some years with favorable conditions oak may turn red in early summer due to formation of lammas shoots. Seedlings of oak almost always develop lammas shoots. Lammas shoot occurrence is partly genetically fixed and essentially involves breaking bud rest.
In the case of oak, the lammas shoots are in many cases longer than the first shoots in spring. The lammas shoots thus act as an insurance policy against insect herbivory in spring, as in spring not all reserves are put into one extension shoot, with the risk of losing all to herbivory.
The formation of an extra shoot in summer leads to more photosynthesis and shoot extension, but it also poses a risk to the tree. In case of a late extension growth of the lammas shoot, damage from early frosts may occur if the shoot has not yet hardened enough. The leaves on lammas shoots of e.g. oak are also susceptible to attack by mildew (Microsphaera alphitoides) due to the large number of mildew spores in the air at the time of sprouting. Because the lammas shoots are on average longer than the spring shoot in for young oaks, a severe mildew infestation can lead to a sharp reduction in height growth and therefore a reduced competitive position. This is one of the reasons why natural regeneration of oak may have a competitive disadvantage in comparison with other species.
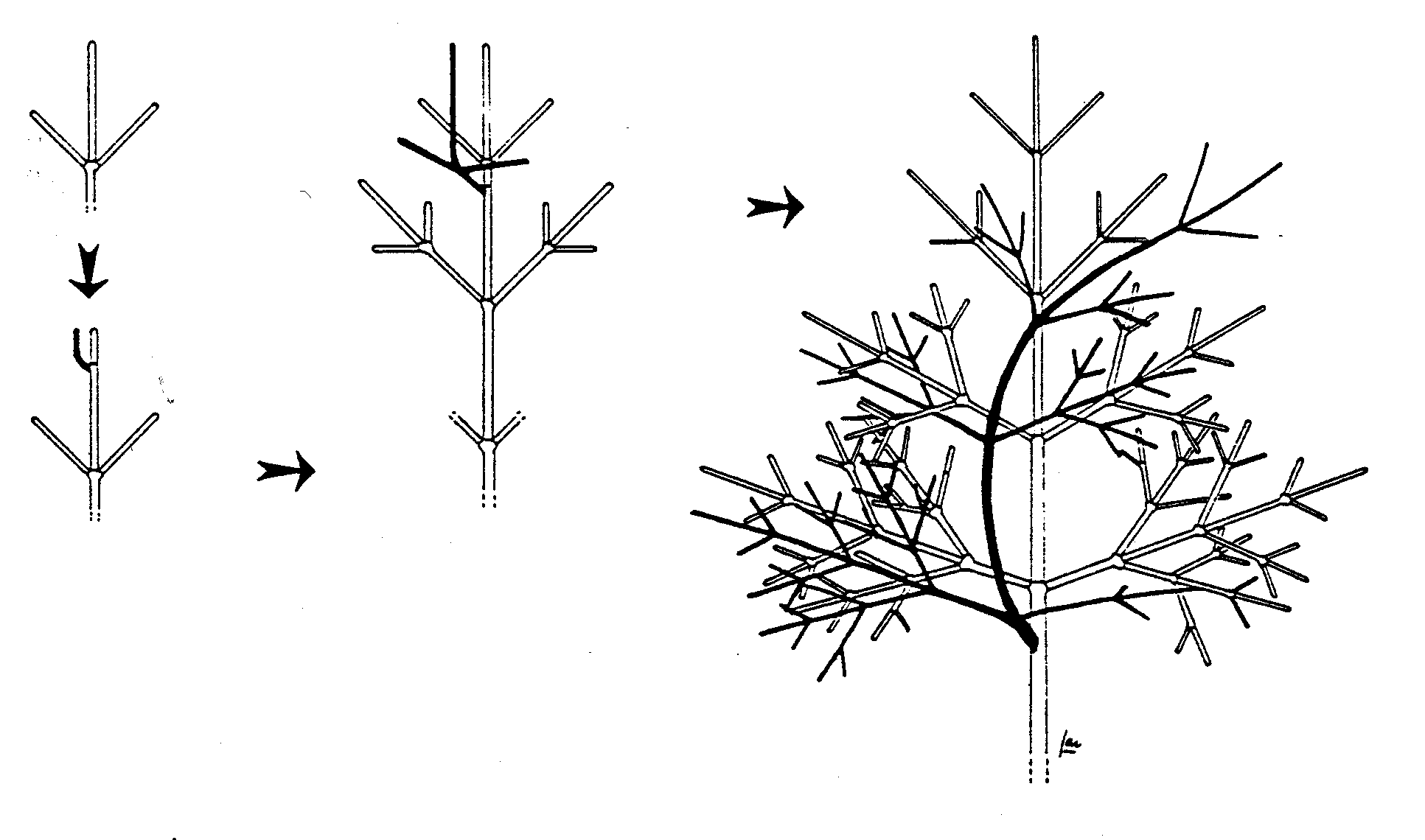
As in the case of oak, lammas shoots in douglas fir are susceptible to fungal infestations. Especially in dense young stands, the lammas shoots may die in large numbers because of encroachment by Botrytis cinerea. The spring shoots are rarely killed by this fungus. Lammas shoots may also lead to large knots in douglas fir, when lammas shoots originate from a side bud, and in the next growing season extend vertically parallel to the top shoot (Fig. B4-1-2). These two shoots can then grow together for a number of years, after which the top shoot may regain apical control. The initially orthotropic growing lammas shoot then suddenly starts to grow plagiotropic and continues to behave like a regular side branch. The result of this is that the Saint John’s shoot is attached to the stem at a very sharp angle and causes a large knot when the tree thickens. This is very unfavourable for wood quality.
 | |
 |  |
Figure B14-1-2. The creation of a double top shoot by the formation of lammas shoot in douglas fir. In black the branch complex that arises from the lammas shoot. The pictures show a lammas shoot adjacent to a lateral bud, and a douglas fir on which a lammas shoot has grown for some time in parallel with the final shoot, after which the direction of extension has been deflected and it has started to grow as a side branch. Illustration and photos: Jan den Ouden.